Joukowski map

The Joukowsky transform is injective on every circle of radius r≠1. Transformada de Joukowski Upiig - IPN - Duration: 9:53. What is Significance of Pole-Zero of Transfer Functions - Duration: 22:16.

TheJoukowskiMappingAirfoilsFromCircles The Wolfram Demonstrations Project contains thousands of free interactive visualizat.

M2Joukowski airfoil ScWpl animation - Duration: 9:06.

University of Technology, Finland.

To transform the disk into an aerofoil shape, I (think!) I need to apply the Joukowski Mapping to an off-centred disk.

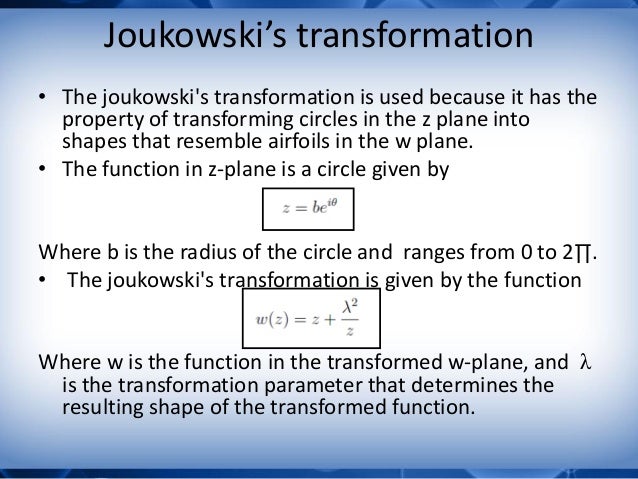
Now to do this, I thought to first apply an affine transformation to . The so called Joukowski function. A closely related conformal mapping , the Kármán–Trefftz transform, generates the much broader class of Kármán–Trefftz airfoils by controlling the trailing edge angle. Near the beginning of the twentieth century Martin Kutta, a German math- ematician, and Nikolay Zhukovski ( Joukowsky in the modern literature), a. I leave it to the reader to verify that the reciprocal of is z. Addition of complex numbers can . Sahoo, Department of Ocean Engineering, IITKharagpur.

Invisci Incompressible Flow Past Circular Cylinders … Fig.

Analysis is effected so as to determine the properties of this transformation function and spheres of both Euclidean and hyperbolic geometry are executed in this expedition. JoukowskiAirfoilFlowField The Wolfram Demonstrations Project contains thousands of free interactive visualizations, with ne. The map is the Joukowski transformation , with the circle . Complex Analysis and Conformal Mapping by Peter J. The classical Joukowski transformation plays an important role in different applications of conformal map - pings, in particular in the study of flows around the so-called Joukowski airfoils.

Barran studied generalized Joukowski transformations of higher order in the complex plane from the view . Conditions that the mapping function be conformal are transplanted from the ellipse to an annulus with the Joukowski map. In addition, the generalized Joukowski transformation is–like in the complex case –an important example for the study of the mapping properties of non-linear monogenic functions. To investigate in more detail some similarities as well as differences between (4) and the complex Joukowski transformation we will continue . By knowing the derivative of the transformation used to perform the geometry mapping, along with the original velocities around the cylinder, the velocities in the mapped flow field can be found.