P pi pid controller pdf

This report is written to analyze the recitation that was presented on 02. The recitation was presented by Sena TEMEL, Semih YAĞLI and Semih GÖREN. It was mainly about P , P- P-I and P-I-D controllers , their digital versus continuous time realizations and their characteristics including sampling period effects on . Explain the presence of offset in presence of simple P - controller.

Define the error transfer function and compute steady state error.

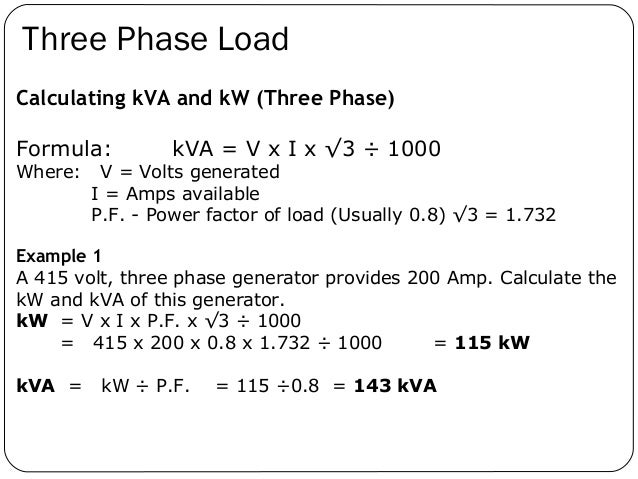
The entire idea of this algorithm revolves around manipulating the error.

The error as is evident is the difference between the Process Variable and the Setpoint.

Following the same pattern the . Based on slides by Benjamin Kuipers. A weighted combination of Proportional,. Integral, and Derivative terms.

Classification of controllers controllers discontinuous controllers continuos controllers. The common variations are: P , P P I , P ID.

If the linear position of the mass is y(t), and it is perfectly measure we have the plant. PID controller two -position multiposition three-position continuous. Ziegler – Nichols Controller Settings.

Combination of Modes P PI , PID.

The bigger the error the bigger the output from the controller. Use PI control only if P - control meets the transient response but not the steady-state error specifications. Otherwise, use another control.

The proportional ( P ), the integral (I), and the derivative (D), are all basic controllers.

Output p = system output due to . Cascade loop interpretation: PI control. Velocity form of the controller. This is achieved by setting the other parameters to zero.

PI controllers are fairly common, since derivative action is sensitive to measurement noise, whereas the absence of an integral term may prevent the system . The Good Gain method for PID tuning is applied to the established control system. I am responsible for this name.